Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF Download

Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF Download: Students of Standard 12 can now download Plus Two Maths Chapter 3 Matrices chapter wise question and answers pdf from the links provided below in this article. Plus Two Maths Chapter 3 Matrices Question and Answer pdf will help the students prepare thoroughly for the upcoming Plus Two Maths Chapter 3 Matrices exams.

Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers

Plus Two Maths Chapter 3 Matrices question and answers consists of questions asked in the previous exams along with the solutions for each question. To help them get a grasp of chapters, frequent practice is vital. Practising these questions and answers regularly will help the reading and writing skills of students. Moreover, they will get an idea on how to answer the questions during examinations. So, let them solve Plus Two Maths Chapter 3 Matrices chapter wise questions and answers to help them secure good marks in class tests and exams.

Board	Kerala Board
Study Materials	Chapter wise Question and Answers
For Year	2021
Class	12
Subject	Mathematics
Chapters	Maths Chapter 3 Matrices
Format	PDF
Provider	Spandanam Blog

How to check Plus Two Maths Chapter 3 Matrices Question and Answers?

1. Visit our website - https://spandanamblog.com
2. Click on the 'Plus Two Question and Answers'.
3. Look for your 'Plus Two Maths Chapter 3 Matrices Question and Answers'.
4. Now download or read the 'Class 12 Maths Chapter 3 Matrices Question and Answers'.

Plus Two Maths Chapter 3 Matrices Question and Answers PDF Download

We have provided below the question and answers of Plus Two Maths Chapter 3 Matrices Chapter wise study material which can be downloaded by you for free. These Plus Two Maths Chapter 3 Matrices Chapter Wise Question and answers will contain important questions and answers and have been designed based on the latest Plus Two Maths Chapter 3 Matrices, books and syllabus. You can click on the links below to download the Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF. 

Question 1.
Find the value of a, b and c from the following equations;
\(\left[\begin{array}{cc}{a-b} & {2 a+c} \\{2 a-b} & {3 c+d}
\end{array}\right]=\left[\begin{array}{cc}{-1} & {5} \\{0} & {13}\end{array}\right]\).
Answer:
Given;
\(\left[\begin{array}{cc}{a-b} & {2 a+c} \\{2 a-b} & {3 c+d}
\end{array}\right]=\left[\begin{array}{cc}{-1} & {5} \\{0} & {13}\end{array}\right]\)
⇒ a – b = -1, 2a + c = 5, 2a – b = 0, 3c + d = 13
⇒ a – b = -1
2a – b = 0
– a = -1
⇒ a = 1
We have, a – b = -1 ⇒ 1 – b = -1 ⇒ b = 2
⇒ 2a + c = 5 ⇒ 2 + c = 5 ⇒ c = 3
⇒ 3c + d = 13 ⇒ 9 + d = 13 ⇒ d = 4.

Question 2.
Simplify cosx\(\left[\begin{array}{cc}{\cos x} & {\sin x} \\{-\sin x} & {\cos x}\end{array}\right]\) + sinx\(\left[\begin{array}{cc}{\sin x} & {-\cos x} \\{\cos x} & {\sin x}\end{array}\right]\).
Answer: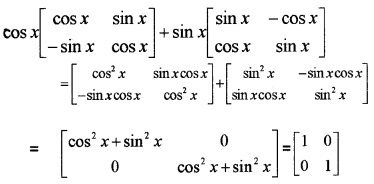

Question 3.
Solve the equation for x, y z and t; if
\(2\left[\begin{array}{ll}{x} & {z} \\{y} & {t}\end{array}\right]+3\left[\begin{array}{cc}{1} & {-1} \\{0} & {2}\end{array}\right]=3\left[\begin{array}{ll}{3} & {5} \\{4} & {6}\end{array}\right]\).
Answer:

⇒ 2x + 3 = 9 ⇒ x = 3
⇒ 2z – 3 = 15 ⇒ z = 9
⇒ 2y = 12 ⇒ y = 6
⇒ 2t + 6 = 18 ⇒ t = 6.

Question 4.
Find A² – 5A + 6I If A = \(\left[\begin{array}{ccc}{2} & {0} & {1} \\{2} & {1} & {3} \\{1} & {-1} & {0}\end{array}\right]\)
Answer:

A² – 5A + 6I

Question 5.
If A = \(\left[\begin{array}{cc}{3} & {-2} \\{4} & {-2}\end{array}\right]\) find k so that A² = kA – 2I.
Answer:

Given A² = kA – 2I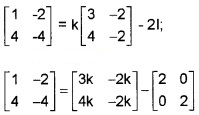

1 = 3k – 2
⇒ k = 1.

Question 6.
Express A = \(\left[\begin{array}{ccc}{-1} & {2} & {3} \\{5} & {7} & {9} \\{-2} & {1} & {1}
\end{array}\right]\) as the sum of a symmetric and skew symmetric matrix.
Answer:

P = 1/2 (A + A^T) is symmetric.
Q = 1/2 (A – A^T) is skew symmetric.

Question 7.
Find the inverse of the following using elementary transformations.

Answer:
(i) Let A = I A

(ii) Let A = IA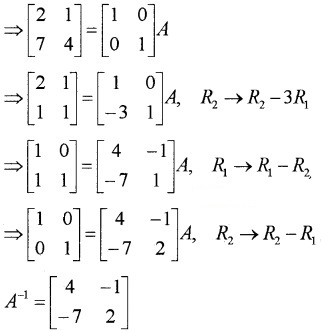

(iii) Let A = IA

(iv) Let A = IA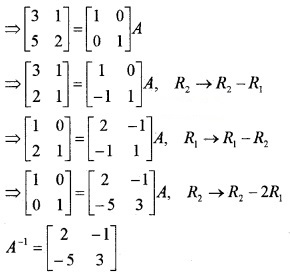

Question 8.
Find the inverse of the matrix A = \(\left[\begin{array}{cc}{2} & {3} \\{-1} & {5}\end{array}\right]\) using row transformation.
Answer:
A = \(\left[\begin{array}{cc}{2} & {3} \\{-1} & {5}\end{array}\right]\)
Let A = IA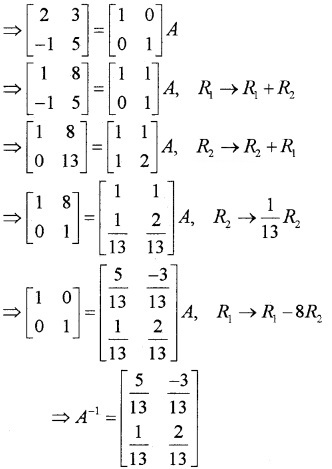

Question 9.
\(A=\left[\begin{array}{ll}{2} & {3} \\{4} & {5} \\{2} & {1}\end{array}\right] B=\left[\begin{array}{ccc}{1} & {-2} & {3} \\{-4} & {2} & {5}\end{array}\right]\)

1. Find AB
2. If C is the matrix obtained from A by the transformation R₁ → 2R₁, find CB

Answer:

(ii) Since C is the matrix obtained from A by the transformation R₁ → 2R₁
⇒ C = \(\left[\begin{array}{ll}{4} & {6} \\{4} & {5} \\{2} & {1}\end{array}\right]\)
Then CB can be obtained by multiplying first row of AB by 2.
CB = \(\left[\begin{array}{ccc}{-20} & {-4} & {42} \\{-16} & {2} & {37} \\{-2} & {-2} & {11}
\end{array}\right]\).

Question 10.
Construct a 3 × 4 matrix whose elements are given by

1. a_y = \(\frac{|-3 i+j|}{2}\) (2)
2. a_ij = 2i – j (2)

Answer: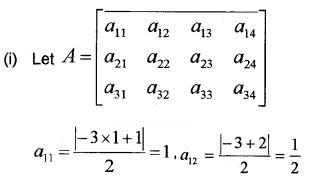

a₁₃ = 0, a₁₄ = \(\frac{1}{2}\), a₂₁ = \(\frac{5}{2}\), a₂₂ = 2, a₂₃ = \(\frac{3}{2}\), a₂₄ = 1, a₃₁ = 4, a₃₂ = \(\frac{7}{2}\), a₃₃ = 3, a₃₄ = \(\frac{5}{2}\)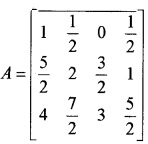

a₁₁ = 1, a₁₂ = 0, a₁₃= -1, a₁₄ = -2, a₂₁ = 3, a₂₂ = 2, a₂₃ = 1, a₂₄ = 0, a₃₁ = 5, a₃₂ = 4, a₃₃ = 3, a₃₄ = 2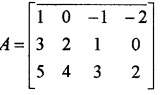

Question 11.
Express the following matrices as the sum of a Symmetric and a Skew Symmetric matrix.
(i) \(\left[\begin{array}{ccc}{6} & {-2} & {2} \\{-2} & {3} & {-1} \\{2} & {-1} & {3}
\end{array}\right]\)
(ii) \(\left[\begin{array}{ccc}{3} & {3} & {-1} \\{-2} & {-2} & {1} \\{-4} & {-5} & {2}
\end{array}\right]\)
Answer: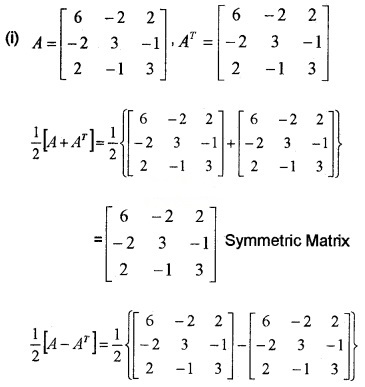

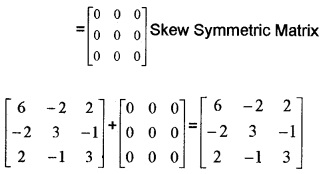
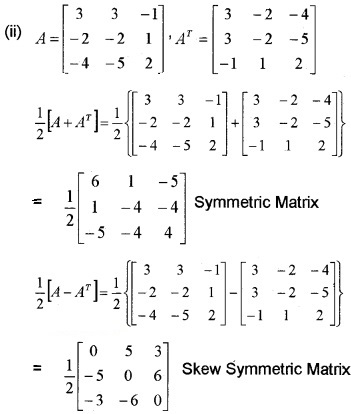
Question 12.
If A = \(\left[\begin{array}{ccc}{2} & {4} & {3} \\{1} & {0} & {6} \\{0} & {-2} & {-3}\end{array}\right]\)

1. Find 3A. (1)
2. Find A^T (1)
3. Evaluate A + A^T , is it symmetric? Justify your answer. (1)

Answer:
1. 3A = \(\left[\begin{array}{ccc}{6} & {12} & {9} \\{3} & {0} & {18} \\{0} & {-6} & {-9}
\end{array}\right]\)

2. A^T = \(\left[\begin{array}{ccc}{2} & {1} & {0} \\{4} & {0} & {-2} \\{3} & {6} & {-3}
\end{array}\right]\)

3. A + A^T

The elements on both sides of the main diagonal are same. Therefore A + A^T is a symmetric matrix.

Plus Two Maths Matrices Four Mark Questions and Answers

Question 1.
Consider the following statement: P(n) : Aⁿ = \(\left[\begin{array}{cc}{1+2 n} & {-4 n} \\{n} & {1-2 n}\end{array}\right]\) for all n ∈ N

1. Write P (1). (1)
2. If P(k) is true, then show that P( k + 1) is also true. (3)

Answer:
1. P(1) : A = \(\left[\begin{array}{cc}{1+2} & {-4} \\{1} & {1-2}\end{array}\right]=\left[\begin{array}{cc}{3} & {-4} \\{1} & {-1}\end{array}\right]\)

2. Assume that P(n) is true n = k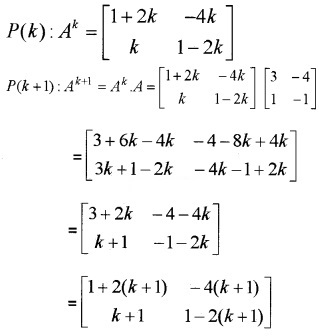

Hence P(k+1) is true n ∈ N.

Question 2.
Find the matrices A and B if 2A + 3B = \(\left[\begin{array}{ccc}{1} & {2} & {-1} \\{0{1} & {2} & {4}\end{array}\right]\) and A + 2B = \(\left[\begin{array}{lll}{2} & {0} & {1} \\{1} & {1} & {2} \\{3} & {1} & {2}\end{array}\right]\).
Answer:

Solving (1) and (2) ⇒ 2 × (2)

Question 3.

1. Construct a 3 × 3 matrix A = [a_ij] where a_ij – 2(i – j) (3)
2. Show that matrix A is skew-symmetric. (1)

Answer:
1.

2.

Therefore A is a skew-symmetric matrix.

Question 4.
Consider the following statement P(n ): Aⁿ = \(\left[\begin{array}{cc}{\cos n \theta} & {\sin n \theta} \\{-\sin n \theta} & {\cos n \theta}\end{array}\right]\) for all n ∈ N

1. Write P(1). (1)
2. If P (k) is true then show that P (k+1) is true (3)

Answer:
1.

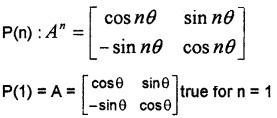
2. Assume that P(n) is true for n = k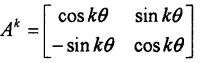

P(k+1) = A^k+1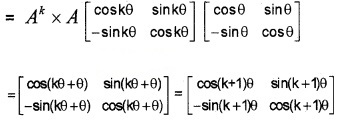

∴ P(k+1) is true. Hence true for all n ∈ N.

Question 5.
A = \(\left[\begin{array}{lll}{1} & {2} & {2} \\{2} & {1} & {2} \\{2} & {2} & {1}\end{array}\right]\), then

1. Find 4A and A² (2)
2. Show that A² -4A = 5I₃ (2)

Answer:
1.

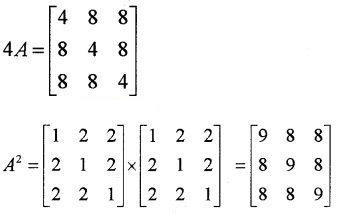
2.

Question 6.
Let A = \(\left[\begin{array}{lll}{2} & {1} & {3} \\{4} & {1} & {0}\end{array}\right]\) and B= \(\left[\begin{array}{cc}{1} & {-1} \\{0} & {2} \\{5} & {0}\end{array}\right]\)

1. Find A^T and B^T (1)
2. Find AB (1)
3. Show that (AB)^T = B^T A^T (2)

Answer:
1.

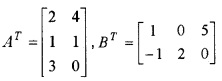
2.

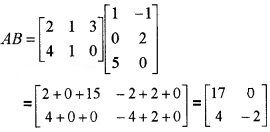
3.

∴ (AB)^T = B^T A^T.

Question 7.
A = \(\left[\begin{array}{ccc}{1} & {-3} & {1} \\{2} & {0} & {4} \\{1} & {2} & {-2}\end{array}\right]\) Express A as the sum of a symmetric and skew symmetric matrix.
Answer: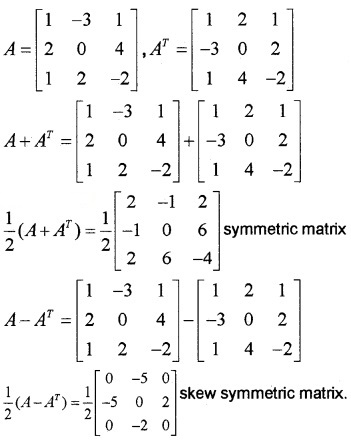

\(\frac{1}{2}\) (A + A^T) + \(\frac{1}{2}\) (A – A^T)

Question 8.

1. Consider a 2 × 2 matrix A = [a_ij], where a_ij = \(\frac{(i+j)^{2}}{2}\)
2. Write the transpose of A. (2)
3. Show that A is symmetric. (2)

Answer:
1. A = \(\left[\begin{array}{ll}{2} & {\frac{9}{2}} \\{\frac{9}{2}} & {8}\end{array}\right]\)

2. A^T = \(\left[\begin{array}{ll}{2} & {\frac{9}{2}} \\{\frac{9}{2}} & {8}\end{array}\right]\)

3. A^T = A therefore symmetric matrix.

Question 9.
A = \(\left[\begin{array}{ll}{6} & {5} \\{7} & {6}\end{array}\right]\) is a matrix

1. What is the order of A. (1)
2. Find A² and 12 A. (2)
3. If f(x) = x^T – 12x +1; find f(A). (1)

Answer:
1. Order of A is 2 × 2.

2.

3. f(x) = x² – 12x + 1 ⇒ f(A) = A² – 12A + I

Plus Two Maths Matrices Six Mark Questions and Answers

Question 1.
Let A = \(\left[\begin{array}{ll}{2} & {4} \\{3} & {2}\end{array}\right]\), B = \(\left[\begin{array}{cc}{1} & {3} \\{-2} & {5}\end{array}\right]\), C = \(\left[\begin{array}{rr}{-2} & {5} \\{3} & {4}\end{array}\right]\)
Find each of the following
(i) A + B; A – B
(ii) 3A – C
(iii) AB
(iv) BA
Answer: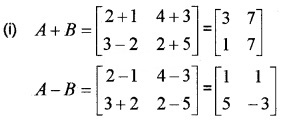

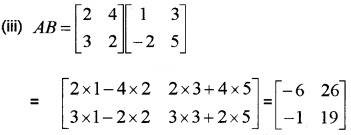
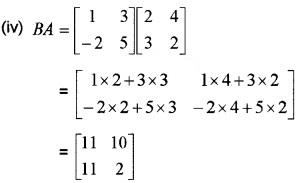
Question 2.
Let A = \(\left[\begin{array}{ll}{1} & {2} \\{3} & {4}\end{array}\right]\); B = \(\left[\begin{array}{ll}{2} & {1} \\{4} & {5}\end{array}\right]\); C = \(\left[\begin{array}{ccc}{1} & {-1} \\{0} & {2}\end{array}\right]\)
(i) Find A + B and A – B (2)
(ii) Show that (A + B) + C = A + (B + C) (2)
(iii) Find AB and BA
Answer:

∴ (A + B) + C = A + (B + C)

Question 3.
A = \(\left[\begin{array}{ccc}{-1} & {0} & {2} \\{4} & {0} & {-3}\end{array}\right]\), B = \(\left[\begin{array}{cc}{0} & {2} \\{-1} & {3} \\{0} & {4}\end{array}\right]\)

1. What is the order of matrix AB ? (1)
2. Find A^T, B^T (2)
3. Verify (AB)^T = B^T A^T (3)

Answer:
1. Order of AB is 2 × 2. Since order of A is 2 × 3 and B is 3 × 2.

2.

3.

(AB)^T = B^T A^T.

Question 4.
Let A = \(\left[\begin{array}{rrr}{1} & {2} & {-3} \\{2} & {1} & {-1}\end{array}\right]\), B = \(\left[\begin{array}{ll}{2} & {3} \\{5} & {4} \\{1} & {6}\end{array}\right]\)
(i) FindAB. (1)
(ii) Find A^T, B^T & (AB)^T (3)
(iii) Verify that (AB)^T = B^T A^T (2)
Answer:

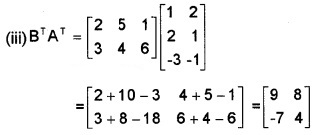
Question 5.
If A = \(\left[\begin{array}{c}{-2} \\{4} \\{5}\end{array}\right]\), B = \(\left[\begin{array}{lll}{1} & {3} & {6}\end{array}\right]\)
(i) Find A^T, B^T (1)
(ii) Find (AB)^T (2)
(iii) Verify (AB)^T = B^T A^T (3)
Answer: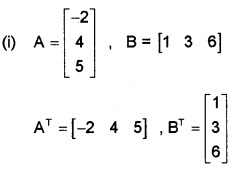

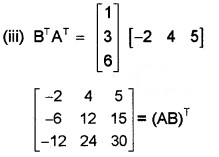
Question 6.
Let A = \(\left[\begin{array}{cc}{3} & {1} \\{-1} & {2}\end{array}\right]\)
(i) Find A² (1)
(ii) Show that A² – 5A + 7I = 0 (1)
(iii) Using this result find A^-1 (2)
(iv) Slove the following equation using matrix: 3x + y = 1, – x + 2y = 2.
Answer:

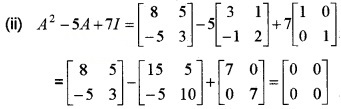
(iii) A² – 5A + 7I = 0 ⇒ A² – 5A = -7I,
multiplying by A^-1 on both sides,
⇒ A – 5I = -7 A^-1

(iv) The equation can be represented in matrix form as follows, AX = B ⇒ X = A^-1B

Question 7.
A = \(\left[\begin{array}{ccc}{1} & {2} & {3} \\{3} & {-2} & {1} \\{4} & {2} & {1}
\end{array}\right]\)
(i) Show that A³ – 23A – 40I = 0 (3)
(ii) Hence find A^-1 (3)
Answer: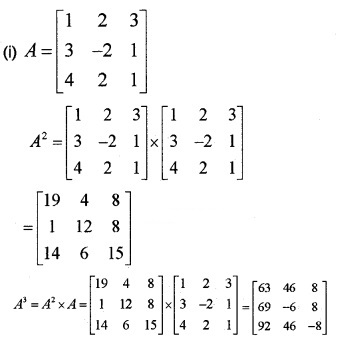

A³ – 23A – 40I = 0

(ii) A^-1A³ – 23 A^-1A – 40A^-1I = 0
⇒ A² – 23I – 40A^-1 = 0

Question 8.
A is a third order square matrix and \(a_{i j}=\left\{\begin{aligned}-i+2 j & \text { if } i=j \\i \times j & \text { if } i \neq j\end{aligned} \text { and } B=\left[\begin{array}{lll}{2} & {1} & {1} \\{1} & {1} & {5} \\{1} & {5} & {2}\end{array}\right]\right.\)

1. Construct the matrix A. (1)
2. Interpret the matrix A. (1)
3. Find AB – BA. (3)
4. Interpret the matrix AB – BA. (1)

Answer:
1. a₁₁ = 1, a₁₂ = 2, a₁₃ = 3, a₂₁ = 2, a₂₂ = 2, a₂₃ = 6, a₃₁ = 3, a₃₂ = 6, a₃₃ = 3
A = \(\left[\begin{array}{lll}{1} & {2} & {3} \\{2} & {2} & {6} \\{3} & {6} & {3}\end{array}\right]\)

2. Now,

Therefore A is symmetric matrix.

3.

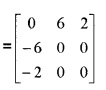
4.

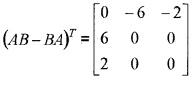
= -(AB – BA)
∴ skew symmetric matrix.

Question 9.
Find x and y if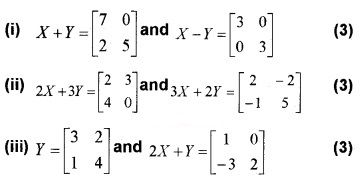

Answer:

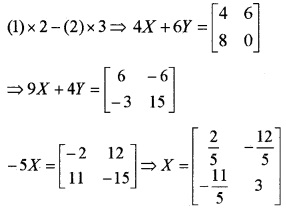
Question 10.
Given that A + B = \(\left[\begin{array}{ll}{2} & {5} \\{7} & {8}\end{array}\right]\) and A – B = \(\left[\begin{array}{ll}{6} & {8} \\{4} & {3}\end{array}\right]\)

1. Find 2A. (1)
2. Find A² – B². (3)
3. Is it equal to (A + B) (A – B)? Give reason (2)

Answer:
1. 2A = A + B + A – B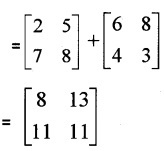

2.

3. (A + B)(A – B)

(A + B)(A – B) = A² + AB – BA – B²
≠ A² – B²
∵ AB ≠ BA.

Question 11.
(i) Consider A = \(\left[\begin{array}{lll}{1} & {x} & {1}\end{array}\right]\), B = \(\left[\begin{array}{ccc}{1} & {3} & {2} \\{2} & {5} & {1} \\{15} & {3} & {2}
\end{array}\right]\), C = \(\left[\begin{array}{l}{1} \\{2} \\{x}\end{array}\right]\) (2)

A – Matrix	B – Order
A	3 × 1
B	1 × 1
BC	2 × 2
ABC	3 × 3
1 × 3

(ii) Find x if ABC = 0 (4)
Answer:
(i)

A – Matrix	B – Order
A	1 × 3
B	3 × 3
BC	3 × 1
ABC	1 × 1

(ii) Given, ABC = 0

⇒ x² + 16x + 28 = 0
⇒ (x + 14)(x + 2) = 0
⇒ x = -14, -2.

Plus Two Mathematics All Chapters Question and Answers

• Plus Two Mathematics Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 2 Inverse Trigonometric Functions Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 4 Determinants Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 5 Continuity and Differentiability Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 6 Application of Derivatives Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 7 Integrals Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 8 Application of Integrals Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 9 Differential Equations Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 10 Vector Algebra Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 11 Three Dimensional Geometry Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 12 Linear Programming Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 13 Probability Chapter Wise Question and Answers PDF

Benefits of the Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF

The Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF that has been provided above is extremely helpful for all students because of the way it has been drafted. It is designed by teachers who have over 10 years of experience in the field of education. These teachers use the help of all the past years’ question papers to create the perfect Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF.

FAQ’s Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF

Where can I download Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF?

You can download Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF for the latest 2021 session.

Can I download Plus Two All subjects Question and Answers PDF?

Yes - You can click on the links above and download subject wise question papers in PDF

Is there any charge for the Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF?

There is no charge for the model papers for you can download everything free