Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF Download

Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF Download: Students of Standard 12 can now download Plus Two Maths Chapter 1 Relations and Functions chapter wise question and answers pdf from the links provided below in this article. Plus Two Maths Chapter 1 Relations and Functions Question and Answer pdf will help the students prepare thoroughly for the upcoming Plus Two Maths Chapter 1 Relations and Functions exams.

Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers

Plus Two Maths Chapter 1 Relations and Functions question and answers consists of questions asked in the previous exams along with the solutions for each question. To help them get a grasp of chapters, frequent practice is vital. Practising these questions and answers regularly will help the reading and writing skills of students. Moreover, they will get an idea on how to answer the questions during examinations. So, let them solve Plus Two Maths Chapter 1 Relations and Functions chapter wise questions and answers to help them secure good marks in class tests and exams.

Board	Kerala Board
Study Materials	Chapter wise Question and Answers
For Year	2021
Class	12
Subject	Mathematics
Chapters	Maths Chapter 1 Relations and Functions
Format	PDF
Provider	Spandanam Blog

How to check Plus Two Maths Chapter 1 Relations and Functions Question and Answers?

1. Visit our website - https://spandanamblog.com
2. Click on the 'Plus Two Question and Answers'.
3. Look for your 'Plus Two Maths Chapter 1 Relations and Functions Question and Answers'.
4. Now download or read the 'Class 12 Maths Chapter 1 Relations and Functions Question and Answers'.

Plus Two Maths Chapter 1 Relations and Functions Question and Answers PDF Download

We have provided below the question and answers of Plus Two Maths Chapter 1 Relations and Functions Chapter wise study material which can be downloaded by you for free. These Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and answers will contain important questions and answers and have been designed based on the latest Plus Two Maths Chapter 1 Relations and Functions, books and syllabus. You can click on the links below to download the Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF. 

Question 1.
Show that the relation R in the set is given by A = {x ∈ Z, 0 = x = 12}
R = {(a,b): a – b is a multiple of 4}
Answer:
Given; R = {(a,b): a – b is a multiple of 4}
Clearly a – a = 0 is a multiple of 4. So
(a, a) ∈ R, hence Reflexive
(a,b) ∈ R ⇒ a -b is a multiple of 4, imply that b – a is a multiple of 4 ⇒ (b, a) ∈ R So Symmetric.
(a, b), (b, c) ∈ R ⇒ a – b and b – c are multiple of 4.
a – b + b – c is a multiple of 4
a – c is a multiple of 4 ⇒ (a, c) ∈ R So Transitive.
Hence R is an equivalence Relation.

Question 2.
Let R be a Relation in the set A = {1, 2, 3, 4, 5, 6} defines as R = {(x,y): y = 2x – 1}

1. Write R in roster form and find it’s domain and range
2. Is R an equivalence relation ? Justify.

Answer:
1. R ={(1, 1), (2, 3), (3, 5)}
Domain = {1, 2, 3} ; Range ={1, 3, 5}

2. Since (2, 2) ∉ R, R is not reflexive
(2, 3) ∈ R but (3, 2) ∉ R
R is not symmetric.
(2, 3) ∈ R, (3, 5) ∉ R but, (2, 5) ∉ R
R is not transitive
∴ R is not an equivalence relation.

Question 3.

Answer:
Given;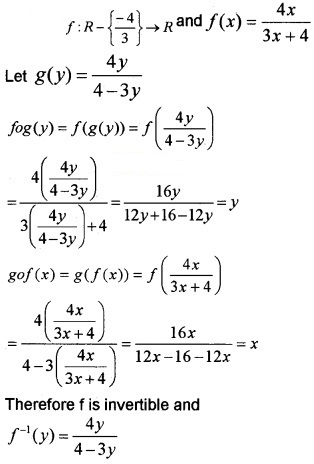

Question 4.
Let ‘ * ’ is a binary operation on the set Q of rational numbers as follows;

1. a * b = a – b
2. a * b = a + ab
3. a * b = a² + b²
4. a * b = (a – b)²

Find which of the binary operation are commutative and associative. Also find the identity element if exists.
Answer:
1. Given; a * b = a – b
b * a = b – a ≠ a – b = a * b
Hence not commutative.
Now; a * (b * c) = a * (b – c)
= a – (b – c) – a – b + c
(a * b) * c = (a – b) * c = a – b – c
a * (b * c) ≠ (a * b) * c
Hence not associative.
Now; a * e = a ⇒ a – e = a ⇒ e = 0
e * a = a ⇒ e – a = a ⇒ e = 2a
a * e ≠ e * a.
So identity element does not exist.

2. Given; a * b = a + ab
b * a = b + ab ≠ a + ab = a * b
Hence not commutative.
Now; a * (b * c) = a * (b + bc)
= a + a(b + be) = a + ab + abc
(a * b) * c = (a + ab) * c
= a + ab + c(a + ab)
= a + ab + ac + abc
a * (b * c) * (a * b) * c
Hence not associative.
Now; a * e = a ⇒ a + ae = a ⇒ e = 0
e * a = a ⇒ e + ea = a ⇒ e = \(\frac{a}{1+a}\)
a * e ≠ e * a.
So identity element does not exist.

3. Given; a * b = a² + b²
b * a = b² + a² = a² + b² = a * b
Hence commutative.
Now; a * (b * c) = a* (b² + c²) = a² + (b² + c²)²
(a * b) * c = (a² + b²) * c
= (a² + b²)² + c²
a * (b * c) ≠ (a * b) * c
Hence not associative.
Now; a * e – a ⇒ a² + e² = a
⇒ e = \(\sqrt{a-a^{2}}\) ∉ Q
So identity element does not exist.

4. Given; a * b = (a – b)²
b * a = (b – a)² = (a – b)² = a * b
Hence commutative.
Now; a * (b * c) = a * (b – c)² (a – (b-c)²)²
(a * b) * c – (a – b)² * c = ((a – b)² – c)²
a * (b * c) ≠ (a * b) * c
Hence not associative.
Now; a * e = \(\sqrt{a}\) ⇒ e = a – \(\sqrt{a}\) ∉ Q
So identity element does not exist.

Question 5.
Show that the relation R on the set of natural numbers defined as R: { (x, y): y – x is a multiple of 2} is an equivalance relation
Answer:
Since x – x = 0 is multiple of 2, (x, x)∈ R
Therefore reflexive.
If y – x is a multiple of 2 then x – y is also a multiple of 2. Therefore (x, y) ∈ R ⇒ (y, x) ∈ R. Hence symmetric.
If y – x is a multiple of 2 and z-y is a multiple of 2, then their sum y – x + z – y = z – x is a multiple of 2. Therefore (x, y), (y, z) ∈ R ⇒ (x, z) ∈ R
Hence transitive.
Therefore R is an equivalance relation.

Question 6.
Consider f : [3, ∞) → [1, ∞) given by f(x) = x² – 6x + 10. Find f^-1
Answer:
f(x) = (x – 3)² + 1
y = (x – 3)² + 1
⇒ (x – 3)² = y – 1
⇒ x = \(\sqrt{y-1}\) + 3
∴ f^-1(x) = \(\sqrt{x-1}\) + 3

Question 7.
‘*’ be a binary operation on N × N defined as (a, b) * (c, d) – (ac, bd)

1. Show that * is commutative.
2. Find the identity element of * if any
3. Write an element of N × N which has an inverse.

Answer:
1. (a, b) * (c, d) = (ac, bd) = (ca, db)
= (c, d) * (a, b)
Hence * is commutative.

2. (a, b) * (e, e) = (a, b)
⇒ (ae, be) = (a, b)
⇒ ae = a ⇒ e = 1
⇒ be = b ⇒ e = 1
Therefore (1,1) is the identity element.

3. Invertible element is (1,1).

Question 8.
Show that the relation R in the set R of Real numbers defined asR = {(a, b): a ≤ b²} is neither reflexive, nor symmetric nor transitive.
Answer:
a ≤ a² is not true for all a. So (a, a) ∉ R hence not Reflexive.
(a, b) ∈ R ⇒ a ≤ b² does not imply that b ≤ a². So (b, a) ∉ R, hence not Symmetric. (a, b),(b, c) ∈ R ⇒ a ≤ b²and b ≤ c² does not imply that a ≤ c². So (a, c) ∉ R, hence not Transitive.

Question 9.
Consider f :R⁺ → [-5, ∞) given by f(x) = 9x² + 6x – 5. Show that fis invertible with f^-1(y) = \(\frac{\sqrt{y+6}-1}{3}\)
Answer:

f is invertible and f^-1(y) = \(\frac{\sqrt{y+6}-1}{3}\).

Question 10.
Let A = R – {3} and B = R – {1} consider the function f: A → B defined by f(x) = \(\frac{x-2}{x-3}\) is f one-one and onto? Justify your answer.
Answer:
Given; f: A → B, A = R – {3} and B = R – {1}
f(x₁) = f(x₂) ⇒ \(\frac{x_{1}-2}{x_{1}-3}=\frac{x_{2}-2}{x_{2}-3}\)
⇒ (x₁ – 2)(x₂ – 3) = (x₂ – 2)(x₁ – 3)
⇒ x₁x₂ – 2x₂ – 3x₁ + 6 = x₂x₁ – 2x₁ – 3x₂ + 6
⇒ -2x₂ – 3x₁ = – 2x₁ – 3x₂ ⇒ x₁ = x₂
Hence one-one
Let y ∈ B ⇒ f(x) = y ⇒ \(\frac{x-2}{x-3}\) = y
⇒ x – 2 = xy – 3y
⇒ x – xy = 2 – 3y
⇒ x(1 – y) = 2 – 3y ⇒ x = \(\frac{2-3 y}{1-y}\) ∈ A=
Hence onto. Therefore bijective.

Question 11.

Answer: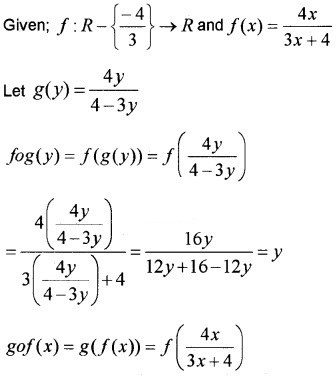

Plus Two Maths Relations and Functions Four Mark Questions and Answers

Question 1.
The relation R defined in the set A = {-1, 0, 1} as R = {(a,b):a = b²}

1. Check whether R is reflexive, symmetric and transitive.
2. Is R an equivalence relation

Answer:
1. (-1, -1) ∉ R, R is not reflexive
(-1,1) ∈ R but (1, -1) ∉ R, R is not symmetric
(-1,1) ∈ R, (1, 1) ∈ R and (-1, 1) ∈ R,
R is transitive.

2. R is not reflexive, not symmetric, and not transitive. So R is not an equivalence relation.

Question 2.
Let A = {1, 2, 3}. Give an example of relation on A which is

1. Symmetric but neither reflexive nor transitive.
2. Transitive but neither reflexive nor symmetric.

Answer:
1. R = {(1, 2),(2, 1)}
(1, 1) ∉ R ⇒ R is not reflexive
(1, 2) ∈ R ⇒ (2, 1) ∈ R, R is symmetric
(1, 2) ∈ R, (2, 1) ∈ R but (1,1) ∉ R,
R is not transitive

2. R = {(1, 2),(1, 3),(2, 3)}
(1, 1) ∉ R ⇒ R is not reflexive
(1, 2) ∈ R ⇒ but (2,1) ∉ R, R is not symmetric
(1, 2) ∈ R, (2, 3) ∈R ⇒ (1, 3) ∈ R,
R is transitive.

Question 3.
Find fog and gof if

1. f(x) – |x| and g(x) – |3x + 4|
2. f(x) = 16x⁴ and g(x) = x ^{\(\frac{1}{4}\)}

Answer:
1. Given; f(x) = |x| and g(x) = |3x + 4|
fog(x) = f(g(x))
= f(|3x + 4|) = ||3x + 4|| = |3x + 4|
gof(x) = g(f(x)) = g(|x|) = |3|x| + 4|

2. fog(x) = f(g(x)) = g(x^{\(\frac{1}{4}\)}) = 16(x^{\(\frac{1}{4}\)})⁴ = x
gof (x) = g(f(x)) = g(16x⁴) = (16x⁴)^{\(\frac{1}{4}\)} = 4x.

Question 4.
Consider the binary operation* : Q → Q where Q is the set of rational numbers is defined as a * b = a + b – ab

1. Find 2 * 3 (1)
2. Is identity for * exist? If yes, find the identity element. (2)
3. Are elements of Q invertible? Is yes, find the inverse of an element in Q (1)

Answer:
1. 2 * 3 = 2 + 3 – 6 = -1.

2. a * e = a ⇒ a + e – ae = a
⇒ e(1 – a) – 0 ⇒ e = 0
e * a = a ⇒ e + a – ea = a
⇒ e(1 – a) = 0 ⇒ e = 0 is the identity element.

3. a * b = 0 ⇒ a + b – ab = 0 ⇒ b = \(\frac{a}{a-1}\)

Question 5.
‘*’ is a binary operation on R defined as a * b = 2ab

1. Determine whether * is commutative and associative
2. Find the identity element if exists.
3. Find the inverse element, if exists

Answer:
1. a * b = 2ab = 2ba = b * a
Therefore commutative
a * (b * c) = a * (2bc) = 4abc
(a * b) * c = (2ab) * c = 4 abc
Therefore is associative.

2. a * e = a ⇒ 2ae = a ⇒ e = \(\frac{1}{2}\)
e * a = a ⇒ 2ea = a ⇒ e = \(\frac{1}{2}\)
Therefore identity element is \(\frac{1}{2}\).

3. a * b = \(\frac{1}{2}\) ⇒ 2ab = \(\frac{1}{2}\) ⇒ b = \(\frac{1}{4a}\), a ≠ 0.

Question 6.

1. If f: R – {2} → R – {2}, defined by f(x) = \(\frac{2 x-3}{x-2}\) then find fof
2. Which of the following satisfies the condition f^-1 ≠ f.

(a) f : R – {0} → R – {0}, f(x) = \(\frac{1}{x}\)
(b) f :R → R, f(x) = -x
(c) f : R – {-1} → R – {-1}, f(x) = \(\frac{x}{x+1}\)
(d) f: R – {2} → R – {2}, f(x) = \(\frac{2 x-3}{x-2}\)

Answer:
1. fof(x) = f(f(x)) = f\(\left(\frac{2 x-3}{x-2}\right)\)

2. Following satisfies the condition f^-1 ≠ f:
(a) f : R – {0} → R – {0}, f(x) = \(\frac{1}{x}\)
(b) f :R → R, f(x) = -x
The graph of functions in (a) and (b) symmetric with respect to the line y = x.
The function in (d) we have already shown that fof (x) = x.
So the answer is (c)
f : R – {-1} → R – {-1}, f(x) = \(\frac{x}{x+1}\).

Question 7.
If f :R → R is a function defined by f(x) = 3x – 2

1. Show that f is one-one.
2. Find fof(x).
3. Find the inverse of f if exists.

Answer:
1. f(x₁) = f(x₂)
⇒ 2x₁ – 3 = 2x₂ – 3 ⇒ x₁ = x₂
Therefore one-one.

2. fof(x) = f(f(x)) = f(3x – 2)
f (x) = 3(3x – 2) – 2 = 9x – 6 – 2 = 9x – 8.

3. Let g(x) = \(\frac{x+2}{3}\)
fog(x) = f(g(x))
= f\(\left(\frac{x+2}{3}\right)\) = 3\(\left(\frac{x+2}{3}\right)\) – 2 = x
Similarly we can show that
gof(x) = g(f(x)) = x
Therefore; f^-1 = \(\frac{x+2}{3}\).

Question 8.
Let A = N × N and be the binary operation. On A defined by (a, b) * (c, d) = (a+c, b+d). Show that ‘ * ’ is commutative and associative. Find the identity for ‘ * ’ on A if any.
Answer:
Given; A = N × N and
(a, b) * (c, d) = (a + c, b + d)
(c, d) * (a, b) = (c + a, d + b)
= (a + c, b + d) = (a, b) * (c, d))
Hence commutative.
Now; (a, b) * [(c, d) * (e, f)] = (a, b) * [c + e, d+f]
= (a + c + e, b + d + f)
[(a, b) * (c, d)] * (e, f) = [a + c, b + d] * (e, f)
= (a + c + e, b + d + f)
Hence associative.
(a, b) * (e, e) = (a, b) ⇒ (a + e, b + e) = (a, b)
⇒ a + e = a, b + e = b ⇒ e=0, e = 0 ⇒ (0,0) ∉ A
So identity element does not exist.

Question 9.
If f(x) = \(\left(\frac{4x+3}{6x-4}\right)\), x ≠ \(\frac{2}{3}\)

1. Show that fof{x)=x, for all x ≠ \(\frac{2}{3}\).
2. What is the inverse of ‘f?

Answer:
1.

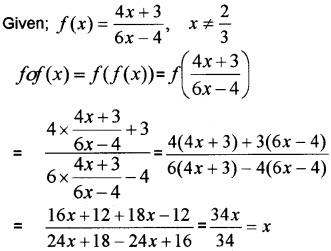
2. Since; fof(x) = x
f is the inverse of itself.

Question 10.
1. Let f be a function defined by f(x) = \(\sqrt{x}\) is a function if it defined from (1)
(a) f : N → N
(b) f : R → R
(c) f : R → R⁺
(d) f : R⁺ → R⁺
2. Check the injectivity and surjectivity of the following functions (3)
(a) f : N → N defined by f(x) = x³
(b) f : R → R given by f(x) = [x]
Answer:
1.
(d) f : R⁺ → R⁺

2.
(a) For x, y ∈ N,
f(x) = f(y) ⇒ x³ = y³ ⇒ x = y
Therefore, f is injective
For 2 ∈ N, there does not exist x in the domain N such that f(x) = x³ = 2.
∴ f is not surjective.

(b) f : R → R given by f(x) = [x]
It seen that f(1.1) = 1 and f(1.8) = 1;
But 1.1 ≠ 1.8;
∴ f is not injective
There does not exist any element x ∈ R
such that f(x) = 0.7
∴ f is not surjective.

Plus Two Maths Relations and Functions Six Mark Questions and Answers

Question 1.
Consider the function f(x) \(=\frac{x+1}{x-1}\); x ≠ 1
1. fof(2) = ____
(a) 1
(b) 2
(c) 3
(d) 4
2. What is the inverse of f?
3. f(3) + f^-1(3)
Answer:
1. fof(2):
(b) 2

2. Let g : range of f → R- {1} inverse of f Let ‘y be any arbitrary element in the range of f
then y = f(x) = \(\frac{x+1}{x-1}\)
⇒ xy – y = x + 1 ⇒ x(y – 1) = y +1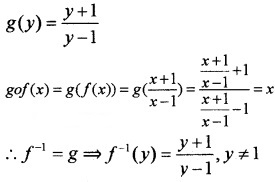

Let us defi ne g : range of f → R- {1} as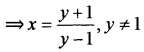

3. f(3) = 2, f^-1(3) = 2
f(3) + f^-1(3) = 2 + 2 = 4.

Question 2.
1. Show that the function f: R → R defined by f(x) = 2x – 3 is one-one and onto. Find f^-1
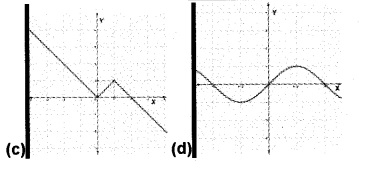
2. Which of the following figure represents the graph of a function on R which is onto but not one-one.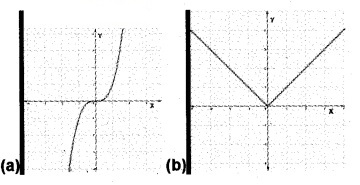


3. Write a function on R which is onto but not one-one
Answer:
1. f(x) = 2x – 3
f(x₁) = f(x₂)
⇒ 2x₁ – 3 = 2x₂ – 3 ⇒ x₁ = x₂
Therefore f is one-one.
Let f(x) = yeR, then
y = 2x – 3 ⇒ 2x = y + 3 ⇒ x = \(\frac{y+3}{2}\) ∈ R
Therefore f is onto. Then f^-1(x) = \(\frac{x+3}{2}\).

2. Option (c)
[(b),(c),(d) are not one-one since for different values of x, we have the same value of y. ie; horizontal line meets at more than one point, (b) and (d) are not onto since range and codomain are different].

3. f(x) = x², f : R → [0, ∞) (Any other function).

Question 3.
A = {1, 2, 3, 4, 6}, * is a binary operation on A is defined as a * b = HCF of a and b.

1. Represent * with the help of an operating table.
2. Find the identity element.
3. Write a commutative binary operation on A with 3 as the identity element. (Hint: Operation table may be used.

Answer:
1.

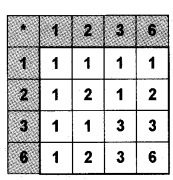
2. From the table it is clear that the identity element is 6.

3. We can write any operation table which is commutative with 3 as the identity element.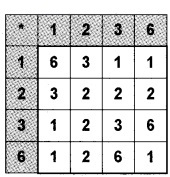

Question 4.
Let * be a binary operation on the set of all real numbers R defined by a * b = a + b + a²b for a, b R.

1. Find 2 * 6 and 6 * 2. (2)
2. Prove that * is neither commutative nor associative. (2)
3. Find the identity elements in R if exists. (2)

Answer:
1. a * b = a + b + a²b
2 * 6 = 2 + 6 + 2² × 6 = 8 + 24 = 32
6 * 2 = 6 + 2 + 6² × 2 = 8 + 72 = 80.

2. a * b = a + b + a²b a * b ≠ b * a
b * a = b + a + b²a
∴ is not commutative.
(ab)c = (a + b + a²b) c
= a + b + a²b + c + (a + b + a²b)²c
a * (b * c) = a * (b + c + b²c)
= a + b + c + b²c + a²(b + c + b²c)
∴ is not associative.

3. Let e is the identity element.
∴ a * e = a ⇒ a + e + a²e = a ⇒ e(1 + a²) = 0
∴ e = 0
∴ e * a = a ⇒ e + a + e²a = a ⇒ e + e²a = 0
⇒ e(1 + ae) = 0
⇒ e = 0; (1 + ae) = 0
ea = -1 ⇒ e = – \(\frac{1}{a}\)
Identity element does not exists.

Question 5.

1. Consider f: {3, 4, 5, 6} → {8, 10, 12, 13, 14} And f = { (3, 8), (4, 10), (5, 12), (6, 14)}. State whether f has inverse ? Give reason. (2)
2. Consider f : R → R given by f(x) = 3x + 2 Show that f is invertible. Find the inverse of f

Answer:
1. Distinct elements in set {3, 4, 5, 6} has distinct images, under f.
∴ f is one- one
But 14 in the codomain has no pre-image.
∴ f is not onto.
∴ f has no inverse.

2. f(x) = 3x + 2; then
f(x₁) = f(x₂) = 3x₂ + 2 ⇒ x₁= x₂
Hence F is one – one
For y ∈ R, let y = 3x + 2 ⇒ x = \(\frac{y-2}{3}\) ∈ R

Plus Two Mathematics All Chapters Question and Answers

• Plus Two Mathematics Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 2 Inverse Trigonometric Functions Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 3 Matrices Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 4 Determinants Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 5 Continuity and Differentiability Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 6 Application of Derivatives Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 7 Integrals Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 8 Application of Integrals Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 9 Differential Equations Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 10 Vector Algebra Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 11 Three Dimensional Geometry Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 12 Linear Programming Chapter Wise Question and Answers PDF
• Plus Two Maths Chapter 13 Probability Chapter Wise Question and Answers PDF

Benefits of the Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF

The Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF that has been provided above is extremely helpful for all students because of the way it has been drafted. It is designed by teachers who have over 10 years of experience in the field of education. These teachers use the help of all the past years’ question papers to create the perfect Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF.

FAQ’s Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF

Where can I download Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF?

You can download Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF for the latest 2021 session.

Can I download Plus Two All subjects Question and Answers PDF?

Yes - You can click on the links above and download subject wise question papers in PDF

Is there any charge for the Plus Two Maths Chapter 1 Relations and Functions Chapter Wise Question and Answers PDF?

There is no charge for the model papers for you can download everything free