Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF Download

Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF Download: Students of Standard 11 can now download Plus One Physics Chapter 3 Motion in a Straight Line question and answers pdf from the links provided below in this article. Plus One Physics Chapter 3 Motion in a Straight Line Question and Answer pdf will help the students prepare thoroughly for the upcoming Plus One Physics Chapter 3 Motion in a Straight Line exams.

Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers

Plus One Physics Chapter 3 Motion in a Straight Line question and answers consists of questions asked in the previous exams along with the solutions for each question. To help them get a grasp of chapters, frequent practice is vital. Practising these questions and answers regularly will help the reading and writing skills of students. Moreover, they will get an idea on how to answer the questions during examinations. So, let them solve Plus One Physics Chapter 3 Motion in a Straight Line questions and answers to help them secure good marks in class tests and exams.

Board	Kerala Board
Study Materials	Question and Answers
For Year	2021
Class	11
Subject	Hindi
Chapters	Physics Chapter 3 Motion in a Straight Line
Format	PDF
Provider	Spandanam Blog

How to check Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers?

1. Visit our website - https://spandanamblog.com
2. Click on the 'Plus One Question and Answers'.
3. Look for your 'Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers'.
4. Now download or read the 'Class 11 Physics Chapter 3 Motion in a Straight Line Question and Answers'.

Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF Download

We have provided below the question and answers of Plus One Physics Chapter 3 Motion in a Straight Line study material which can be downloaded by you for free. These Plus One Physics Chapter 3 Motion in a Straight Line Question and answers will contain important questions and answers and have been designed based on the latest Plus One Physics Chapter 3 Motion in a Straight Line, books and syllabus. You can click on the links below to download the Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF. 

Question 1.
Which of the following curves does not represent motion in one dimension?

Answer:
(b) In one-dimensional motion, the body can have at a time one value of velocity but not two values of velocities.

Question 2.
Free fall of an object (in vacuum) is a case of motion with
(a) Uniform velocity
(b) Uniform acceleration
(c) Variable acceleration
(d) Uniform speed
Answer:
(b) Uniform acceleration:
Free fall of an object (in vacuum) is a case of motion with uniform acceleration.

Question 3.
The area under velocity-time graph fora particle in a given interval of time represents
(a) velocity
(b) acceleration
(c) work done
(d) displacement
Answer:
(d) displacement:
Area under velocity-time graph represents displacement of a particle in a given interval of time.

Question 4.
The velocity-time graph of a body moving in straight line is shown in the figure. The displacement and distance travelled by the body in 6s are respectively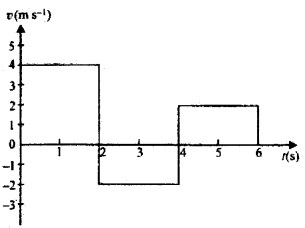

(a) 8, 16m
(b) 16m, 8m
(c) 16m, 16m,
(d) 8m, 8m
Answer:
(a) 8, 16m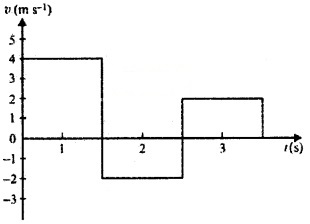

Displacement is equal to area under the velocity-time graph with proper sign.
∴ Displacement = 4 × 2 – 2 × 2 + 2 × 2 = 8m
Distance is equal to total area under the speed time graph.
∴ Distance = 4 × 2 + 2 × 2 + 2 × 2 = 16m.

Question 5.
A cartravels half the distance with constant velocity of 40 kmph and the remaining half with a constant velocity 60 kmph. The average velocity of the car in kmph is
(a) 40
(b) 45
(c) 48
(d) 50
Answer:
(c) 48
Average velocity = ν_av = \(\frac{s}{\frac{s}{80}+\frac{s}{120}}\) = 48kmph.

Question 6.
Two objects A and B travel from P to Q through two different paths as shown in figure. If both A and B takes the same time interval to travel from P to Q, then which of the following statements are true?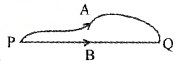

(a) A and B have same speed.
(b) A and B have same velocity
(c) A and B have same average velocity.
(d) The speed of A is greater than that of B
(e) The speed of B isgreaterthanthatofA
Answer:
(d) The speed of A is greater than that of B.

Question 7.
The acceleration of a moving object is equal to the
(a) gradient of a displacement-time graph
(b) gradient of a velocity-time graph
(c) area below a speed-time graph
(d) area below a displacement – time graph
(e) area below a velocity-time graph
Answer:
(b) Gradient of a velocity-time graph.

Question 8.
A ball is thrown vertically upwards and comes back. Which of the following graph represents the velocity-time graph of the ball during its flight?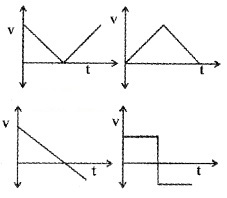

Answer: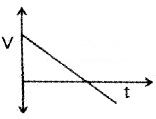

Question 9.
The magnitude of average velocity is equal to average speed. In which case this condition is satisfied?
Answer:
When a particle is moving with constant velocity, the magnitude of its average velocity is equal to average speed.

Question 10.
Can a body be said to be at rest as well as in motion at the.same time?
Answer:
Yes, rest and motion are relative terms. A body at rest with respect to one body may be in motion with respect to another body.

Question 11.
What conclusion can you draw if the average velocity is equal to instantaneous velocity?
Answer:
The particle is moving with constant velocity.

Question 12.
Two cars are moving in such a way that their relative velocity is zero. Which of the following graph represent this situation?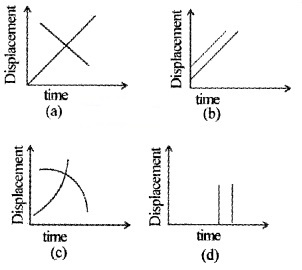

Answer:
(a) b

Question 13.
The speed-time graph is shown in figure. Is it possible.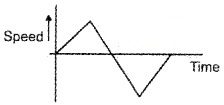

Answer:
No speed cannot be negative.

Question 14.
Why the speed of the object can never be negative?
Answer:
Speed is distance covered per unit time. Since distance cannot be negative, speed cannot be negative.

Question 15.
Is it possible that the velocity of an object be in a direction other than the direction of acceleration? If yes, give an example.
Answer:
Yes. A body is moving with decreasing velocity.

Question 16.
Is it possible to have the rate of change of velocity constant while the velocity itself changes both in magnitude and direction? If yes, give an example.
Answer:
Yes. Projectile motion.

Question 17.
If the acceleration of the particle is constant in magnitude but not in direction, what type of path does the body flow?
Answer:
Circular path.

Question 18.
Two stones of different sizes are dropped simultaneously from the top of a building. Which stone would reach earlier? Why?
Answer:
Both reach at ground simultaneously. Acceleration is same for both stones.

Question 19.
A piece of paper and iron piece are dropped simultaneously from the same point in vacum. Which one will reach at ground earlier?
Answer:
Both reach at ground simultaneously.

Question 20.
Is it possible that your cycle has a southward velocity but northward acceleration? If yes, give an example.
Answer:
Yes, when brakes are applied to a moving cycle, the directions of velocity and acceleration becomes opposite.

Plus One Physics Motion in a Straight Line Two Mark Questions and Answers

Question 1.
An ant is moving through a graph paper along x-axis. A boy observes that the ant covers 1mm in every second.

1. What type of motion is this?
2. When the boy is in school bus he observe the speedometer of the bus. Which speed is observed by the speedometer?

Answer:

1. Uniform motion or uniform velocity
2. Instantaneous speed (Ratio of the displacement to small interval of time).

Question 2.
Match the following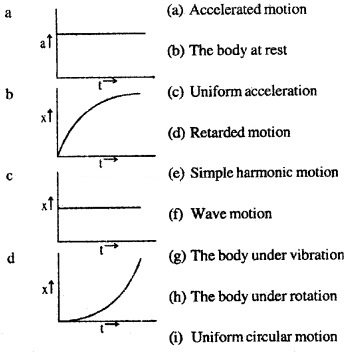

Answer:
a. (c)
b. (d)
c. (b)
d. (a)

Question 3.
Some examples of motion are given below. State in each case if the motion is one, two or three dimension

1. A Kite flying on a windy day.
2. A speeding car on a long straight highway.
3. A carrom coin rebounding from the side of the board.
4. A planet revolving around its star.

Answer:

1. 3 Dimensional motion
2. 1 Dimensional motion
3. 2 Dimensional motion
4. 2 Dimensional motion

Plus One Physics Motion in a Straight Line Three Mark Questions and Answers

Question 1.
Two bodies start moving in the same straight line at the same instant of time from the same origin. The first body moves with a constant velocity of 40 m/s and the second starts from rest with a constant acceleration of 4 m/s².

1. What is uniform speed?
2. Find the time that elapses before the second catches the first body.

Answer:
1. A body is said to be uniform if it travels equal displacements in equal intervals of time.

2. Distance travelled by first body in a time t
S₁ = Vt
S₁ = 40 × t
Distance travelled by second body in a time t
S₂ = ut + \(\frac{1}{2}\) at²
S₂ = \(\frac{1}{2}\) × 4 × t²
When these two bodies meet,
S₁ = S₂
40 × t = \(\frac{1}{2}\) × ²
t = 20 s.

Question 2.
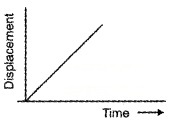
Velocity time graph of a moving object is shown below.

1. What is the acceleration of the object?
2. Draw displacement – time graph for the above motion shown in the graph.

Answer:
1. Acceleration = 0

2.

Question 3.
Two straight lines drawn on the same displacement time graph make angles 30° and 60° with time axis respectively in the figure.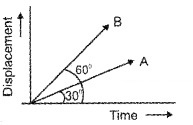

1. Which line represents greater velocity?
2. What is the ratio of the velocity of line A to the velocity of line B?

Answer:
1. B

2.

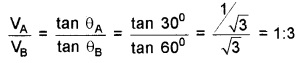
Question 4.
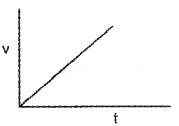
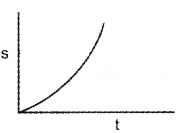
A particle starts from rest and its acceleration plotted against time t is shown below.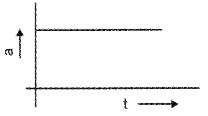

1. This body is at
   • constant acceleration
   • variable acceleration
   • constant velocity
   • rest
2. Plot the corresponding velocity (V) against time (t)
3. Plot the corresponding displacement (S) against time (t)

Answer:
1. Constant acceleration

2.

3.

Question 5.
Displacement is a vector quantity which distance is a scalar quantity.

1. Distinguish between scalar and vector quantities.
2. An athlete runs along a circular track of radius 50m. Find the distance travelled and the displacement of the athlete when he coveres % of the circle.
3. What is the distance travelled by a body in a time t having an initial velocity u and moving with uniform acceleration ‘a’?

Answer:
1. A physical quantity having both magnitudes and direction is called vecter. A physical quantity having only magnitude is called scalar.

2.

Displacement AD

Distance, ABCD = AB + BC + CD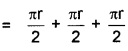

Distance = \(\frac{3}{2}\) πr

3. c = ut + \(\frac{1}{2}\) at²

Question 6.
“The aerial distance between two towers is 4km. But speedometer of car shows 5.6km when travel from one tower to another”

1. By reading this statement explain the concept of distance and displacement.
2. What is the numerical ratio of displacement of object to distance? Explain.
3. A particle is moving along a circular trace of radius ‘R’. What is the distance travelled and displacement of the particle in half revolution?

Answer:
1. Distance os the length of the path covered by the object. It is a scalar quantity. Displacement is the length between initial point and final point.

2. \(\frac{\text { displacement }}{\text { distance }} \leq 1\)
For the straight-line path, displacement is equal to the distance travelled. But for the curved path displacement is less than the distance travelled.

3. distance = πR
displacement = R + R = 2R.

Plus One Physics Motion in a Straight Line Four Mark Questions and Answers

Question 1.
A stone is thrown upwards from the ground with a velocity ‘u’.

1. What is the maximum height attained by the stone?
2. Check the correctness of the equation obtained in (a) using the method of dimensional analysis.
3. Draw the position-time graph of the stone during its return journey. (g = 10m/s²)

Answer:
1. V² = u² + 2as
0 = u² + 2gH
H = u²/2g

2. H = \(\frac{u^{2}}{2 g}\)
When we write the above equation in terms of dimension, we get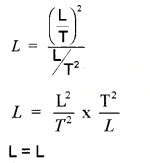

This means that, H= \(\frac{u^{2}}{2 g}\) is dimensionally correct.

3.

Question 2.
Gopal dropped an apple from the top of his flat at a height of 10m. He told his sister Seetha on the ground below that it will reach the ground in 2 seconds after he drops it.

1. Can she catch it after 2 seconds?
2. Derive suitable relation for time of fall.
3. Draw the velocity-time graph of the above body (assume the body rebounds from the floor)

Answer:
1. No.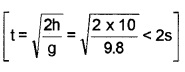

2. S = ut + 1/2at²
h = 0 + 1/2gt²
If ball is dropped from a height ‘h’, we can write.
\(\sqrt{\frac{2 h}{g}}\) = t.

3.

Question 3.
A car of mass 1000 kg starts from rest at t = 0 and under goes acceleration as shown in figure.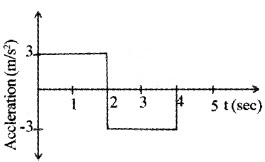

1. Draw the corresponding velocity-time graph.
2. What is the retarding force acting on the car?
3. What is the total distance travelled by the car during t = 0 to t = 4 sec.

Answer:
1.

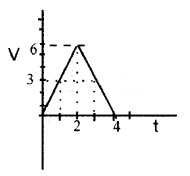
2. Retarding acceleration, a = -3m/s²
∴ Retarding force F = ma
= 1000 × 3 = 3000N.

3. Area of velocity time gives distance.
∴ Area = \(\frac{1}{2}\)bh = \(\frac{1}{2}\) × 4 × 6
Distance = 12m.

Question 4.
A tow rope used to pull the car of mass 700kg will break if the tension exceeds 1500N.

1. Calculate the maximum acceleration with which the car can be pulled through a level road
2. Calculate the minimum time required to bring the car to work station 500m away from the break point

Answer:
1. T = ma
1500= 700 × a
a = \(\frac{1500}{700}\) = 2.14 m/s².

2. S = ut + 1/2 at²
500= 0 + 1/2 × (2.14) × t²
t = \(\sqrt{\frac{2 \times 500}{2.14}}\) = 21.61sec.

Question 5.
1. Figure shows the position-time graph of the one-dimensional motion of a particle. Is it correct to say from the graph that the particle moves in a straight line for t ≤ 0 and on a parabolic path fort> 0? Justify your answer.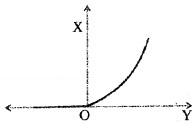

2. Can a body have an acceleration without velocity? Justify your answer with a physical situation.
3. The table given below shows the velocity of a car at different times.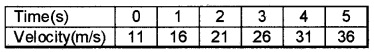

a. Draw acceleration time graph
b. Find the distance traveled by car in 6 sec.
Answer:
1. No. initially the body remains at rest and then the body moves with constant acceleration in a straight line.

2. Yes, consider the oscillation of simple pendulum. At extreme position, velocity becomes zero and acceleration is non – zero value.

3. The velocity of a car at different times:
a. acceleration a = \(\frac{16-4}{1-0}\) = 5 m/s²
This value (acceleration is constant through-out the motion. Hence the acceleration time graph will be a straight line parallel to time axis.

b. S = ut + \(\frac{1}{2}\) + 2at²
= 11 × 6 + \(\frac{1}{2}\) × 5 × 6²
S = 156m.

Question 6.
The relative velocity of body A with respect to a body B is the time rate at which body A changes its position with respect to body B.

1. If V_A and V_B are the velocities of A and B moving in opposite directions, what is the relative velocity of A with respect to B?
2. Two trains along the same straight rails are moving with a constant velocity of 60 km/h and 30 km/b towards each other. If at time t = 0, the distance between them is 90km, find the time when they collide.
3. The velocity-time graph of two bodies A and B make angles of 30° and 60° with the time axis, what is the ratio of their acceleration?

Answer:
1. V_BA = V_A + V_B

2. Relative velocity = 60 + 30
= 90km/h
∴ Hence t = \(\frac{\text { displacement }}{\text { relative velocity }}\)
= \(\frac{90}{90}\) = 1h.

3. Slope of velocity time graph gives acceleration. Hence

Plus One Physics Motion in a Straight Line Five Mark Questions and Answers

Question 1.
A balloon is ascending at the rate of 14 ms^-1 and at a height of 98 m above the ground. A stone is dropped from it.

1. State whether the motion of the balloon is accelerated or retarded.
2. After how much time does the stone reach the ground?
3. Determine the velocity with which the stone strikes the ground.

Answer:
1. The motion of balloon is uniform motion. It has neither acceleration nor retardation.

2. u = 14m/s, a = -9.8, S = -98 m.
S = ut + \(\frac{1}{2}\) at²
98 = 14 t – \(\frac{1}{2}\) × 9.8 t²
4.9 t² – 14 t – 98 = 0
Solvingthisweget t = 6.123 sec

3. ν² = u² + 2as
^ν2 = (14)² + 2 x ^–9.8 x ^–98
= 196 + 1920.8
^ν2 = 2116.8
^ν2 = \(\sqrt{2116.8}\) = 45.99 m/s.

Question 2.
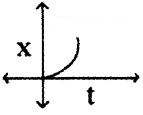
A particle is moving along the x-axis with uniform positive acceleration.

1. Draw the position-time graph for its motion.
2. Obtain the expression for the displacement by drawing a velocity-time graph.
3. A ball is thrown vertically upwards with a velocity of 20 ms^-1 from the top of the tower of height 25m from the ground. How long does it remain in the air? (g = 10 ms^-2)

Answer:
1.

2.

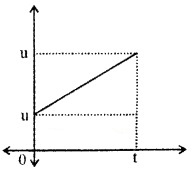
Consider a body moving with an acceleration ‘a’. Let ‘u’ be the initial velocity at t = 0 and final velocity ‘v’ at t = t. The area of the velocity-time graph gives the displacement. This is a quadratic equation. Hence t can be found using this formula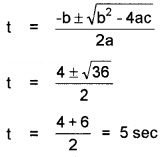

Question 3.

1. State the difference between speed and velocity. Can a body move with uniform speed but with variable velocity? Explain with the help of an example.
2. Show that a body thrown vertically upwards returns with the same magnitude of velocity.

Answer:
1. Speed is a scalar quantity but velocity is a vector quantity. If a body moving along the circumstance of a circle with uniform speed, its velocity changes continuously with time.

2.

Consider a body projected upward from a point A with a velocity ‘u’. If the body reaches at B, the displacement becomes zero. Hence time taken to reach at B can be found.
S = ut + 1/2 at²
0 = ut + 1/2 × ^–10 t²
ut = 5t²
t = \(\frac{u}{5}\) ____(1)
The velocity at B can be found using the formula
V_B = u + at _____(2)
substitute eq(1) in eq(2)
V_B = u + ^–10\(\frac{u}{5}\)
= u – 2u
V_B = -u
∴ V_A = -V_B

Plus One Physics Motion in a Straight Line NCERT Questions and Answers

Question 1.
In which of the following examples of motion, can the body be considered approximately a point object:
(a) a railway carriage moving without jerks between two stations.
(b) a monkey sitting on top of a man cycling smoothly on a circular track.
(c) a spinning cricket ball that turns sharply on hitting the ground.
(d) a tumbling beaker that has slipped off the edge of a table.
Answer:
(a) (b)

Question 2.
The position time (x-t) graphs for two children A and B returning from their school O to their homes P and Q respectively are shown in the following figure Choose the correct entries in the brackets as follows: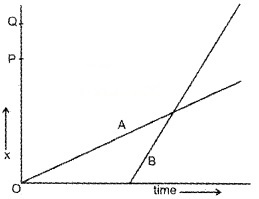

1. (A/B) lives closer to the school than (B/A)
2. (A/B) starts from the school earlier than (B/A)
3. (A/B) walks faster than (B/A)
4. A and B reach home at the (same/different) time
5. (A/B) overtakes (B/A) on the road (once/twice).

Answer:
1. It is clear from the graph that OQ > OP. So, A lives closer to the school than B.

2. The position-time graph of A starts from the origin (t = 0) while the position-time graph of B starts from C which indicates that B started later than A after a time interval OC. So, A started earlier than B.

3. The speed is represented by the steepness (or slope) of the position-time graph. Since the position-time graph of B is steeper than the position-time of graph A, therefore, we conclude that B is faster than A.

4. Corresponding to both P and Q, the time interval is the same, i.e., OD. This indicates that both A and B reach their homes at the same time.

5. The position-time graphs intersect at the point K. This indicates that B crosses A. Since there is only one point of intersection, therefore, the two cyclists cross each other only once.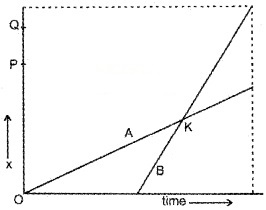

Question 3.
A car moving along a straight highway with speed of 126 kmh^-1 is brought to a stop within a distance of 200m. What is the retardation of the car (assumed uniform), and how long does it take for the car to stop?
Answer:
Initial velocity,
u = 126kmh^-1 = 126 × \(\frac{5}{18}\) ms^-1 = 35m^-1
Final velocity, ν = 0;
Distance, S = 200m
Using ν² – u² = 2aS,
0² – 35 × 35 = 2a × 200
or
a = \(-\frac{35 \times 35}{400}\)ms^-2
= 3.06ms^-2
So, the retardation of the car is 3.06 ms^-2
Using ν = u + at,
0 = 35-3.06 × t
or
3.06t = 35
t = \(\frac{35}{3.06}\)s = 11.4s.

Question 4.
On a two-lane road, car A is travelling with a speed of 36kmh^-1 Two cars B and C approach car A in opposite directions with a speed of 54 kmh^-1 each. At a certain instant, when the distance AB is equal to AC, both being I km, B decides to overtake A before C does. What minimum acceleration of car B is required to avoid an accident?
Answer:
ν_A = 36kmh^-1
= 36 × \(\frac{5}{18}\)ms^-1 = 10ms^-1
ν_B = ν_C = 54ms^-1
= 54 × \(\frac{5}{18}\)ms^-1 = 15ms^-1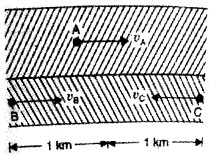

Relative velocity of B w.r.t. A, ν_BA = 5 ms^-1
Relative velocity of C w.r.t.A, ν_CA= 25ms^-1
Time taken by C to cover distance AC
= \(\frac{1000 m}{25 m s-1}\) = 40s
Now, forB, 1000 = 5 × 40 + \(\frac{1}{2}\)a × 40 × 40
On simplification, a = 1 ms^-2.

Question 5.
Read each statement below carefully and state with reasons and examples, if it is true or false; A particle in one-dimensional motion.

1. with zero speed at an instant may have non-zero acceleration at that instant,
2. with zero speed may have non-zero velocity,
3. with constant speed must have zero accelera¬tion,
4. with positive value of acceleration must be speed-ing up.

Answer:

1. True
2. False
3. True
4. False

For (1), consider a ball thrown up. At the highest point, speed is zero but the acceleration is non-zero, For (2), if a particle has non-zero velocity, it must have speed, For (3), if the particle rebounds instantly with the same speed, it implies infinite acceleration which is physically impossible. For (4), true only when the chosen positive direction is along the direction of motion.

Question 6.
A man walks on a straight road from his home to a market 2.5 km away with a speed of 5kmh^-1. Finding the market closed, he instantly turns and walks back home with a speed of 7.5kmh^-1. What is the magnitude of average velocity, and average speed of the man over the following intervals of time:

1. 0 to 30 min,
2. 0 to 50 min,
3. 0 to 40 min?

Answer:
Average speed overthe interval of time from 0 to 30min
= \(\frac{2.5 \mathrm{km}}{30 \mathrm{min}}=\frac{2.5 \mathrm{km}}{\frac{1}{2} \mathrm{h}}\)
= 5kmh^-1
Magnitude of average velocity overthe interval of time from 0 to 30 min is 5kmh^-1. This is because the “distance travelled” and the ‘magnitude of displacement’ over the interval of time from 0 to 30 min are equal. Distance covered from 30 to 50 minutes
= 7.5kmh^-1 × \(\frac{20}{60}\) h = 2.5km
Total distance covered from 0 to 50 minute
= 2.5 km+ 2.5 km = 5km
Total time = 50min = \(\frac{50}{60}\) h = \(\frac{5}{6}\) h
Average speed overthe interval of time from 0 to 50min
= \(\frac{5 \mathrm{km}}{5 / 6 \mathrm{h}}\) = 6kmh^-1
The displacement overthe interval of time from 0 to 50 min is zero. So the magnitude of average velocity is zero. Distance covered from 30 to 40 min
= 7.5kmh^-1 × \(\frac{1}{6}\) h = 1.25km
Total distance covered from 0 to 40 minute
= 2.5 km + 1.25 km = 3.75km
Average speed overthe interval of time from 0 to 40min
= \(\frac{3.75 \mathrm{km}}{\frac{40}{60} \mathrm{h}}\) = 5.625kmh^-1
The “magnitude of displacement” is (2.5 -1.25) km, i.e., 1.25 km.
Time interval = \(\frac{2}{3}\)h
The ‘magnitude of average velocity’ = \(\frac{1.25 \mathrm{km}}{2 / 3 \mathrm{h}}\),
= 1.875 kmh^-1.

Question 7.
Look at the graphs (a) to (d) in the following figure carefully and state, with reasons, which of these cannot possibly represent one-dimensional motion of the particle.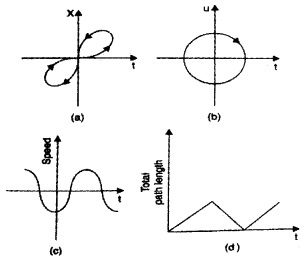

Answer:
None of the four graphs can represent one-dimensional motion of the particle. In fact, all the four graphs are impossible.

1. A particle cannot have two different positions at the same time.
2. A particle cannot have velocity in opposite directions at the same time.
3. Speed is always positive (non-negative).
4. Total path length of a particle can never decrease with time.

Note: The arrows on the graphs are meaningless.

Question 8.
The x-t plot of one-dimensional motion of a particle. Is it correct to say from the graph that the particle moves in a straight line for t < 0 and on a parabolic path for t > 0? If not, suggest a suitable physical context for this graph.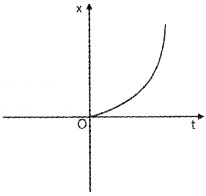

Answer:
No, wrong, x-t plot does not show the trajectory of a particle. Context: A body is dropped from a tower (x = 0) at t = 0.

Question 9.
The velocity-time graph of a particle in one-dimensional motion is shown below. Which of the following formulae are correct for describing the motion of the particle over the time interval from t₁ to t₂?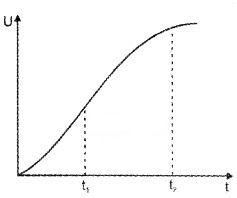

(a) x(t₂) = x(t₁) + ν(t₁)(t₂ – t₁) + \(\frac{1}{2}\) a(t₂ – t₁)²
(b) ν(t₂) = ν(t₁) + a(t₂ – t₁)
(c) ν_average = [x(t₂) – x(t₁)]/(t₂ – t₁)
(d) a_average = [ν(t₂) – ν(t₁)]/(t₂ – t₁)
(e) x(t₂) = x(t₁)+ ν_av (t₂ – ₁) +\(\frac{1}{2}\) a_av(t₂ – t₁)²
(f) x(t₂) – x(t₁) = Area underthe ν – 1 curve bounded by t-axis and the dotted lines.
Answer:
(c), (d), (f)
Explanation: It is clear from the shape of ν – t graph that acceleration of the particle is not uniform between time intervals t₁ and t₂. [Note that the given ν – t graph is not straight.] The equations (a), (b) and (e) represent uniform acceleration.

Plus One Physics All Chapters Question and Answers

• Plus One Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 1 Physical World Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 2 Units and Measurement Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 3 Motion in a Straight Line Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 4 Motion in a Plane Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 5 Law of Motion Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 6 Work, Energy and Power Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 7 Systems of Particles and Rotational Motion Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 8 Gravitation Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 9 Mechanical Properties of Solids Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 10 Mechanical Properties of Fluids Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 11 Thermal Properties of Matter Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 12 Thermodynamics Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 13 Kinetic Theory Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 14 Oscillations Chapter Wise Question and Answers PDF
• Plus One Physics Chapter 15 Waves Chapter Wise Question and Answers PDF

Benefits of the Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF

The Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF that has been provided above is extremely helpful for all students because of the way it has been drafted. It is designed by teachers who have over 10 years of experience in the field of education. These teachers use the help of all the past years’ question papers to create the perfect Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF.

FAQ’s Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF

Where can I download Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF?

You can download Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF for the latest 2021 session.

Can I download Plus One All subjects Question and Answers PDF?

Yes - You can click on the links above and download subject wise question papers in PDF

Is there any charge for the Plus One Physics Chapter 3 Motion in a Straight Line Question and Answers PDF?

There is no charge for the model papers for you can download everything free